Before looking at vertically opposite angles, it’s important to first understand what complementary and supplementary angles are.
Complementary Angles
Complementary angles are 2 angles where when they are added together, they sum up to 90°.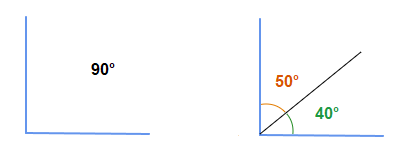
40° and 50° are complementary to each other as angles, as they add up to 90°.
The 2 angles concerned don’t always have to be adjacent, where the angles share a common point/vertex along with a common side between them.
As well as being presented along side each other. Complementary and Supplementary angles can also be apart from each other, thus sharing no point/vertex or side.
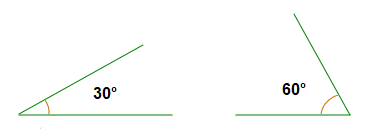
30° and 60° are complementary to one another also. 30° + 60° = 90
Example
(1.1)
What size of angle is complementary to 56°?
Solution
90° − 56° = 34° , 34° + 56° = 90°
The angle 34° is complementary with the angle 56°.
Supplementary Angles
The idea of supplementary angles is quite similar to complementary angles.
Supplementary angles are angles that when added together they sum up to 180°.

130° and 50° are angles that are supplementary. 130° + 50° = 180°
Like in the case of complimentary angles, supplementary angles don’t have to be alongside each other, but at times they can be.
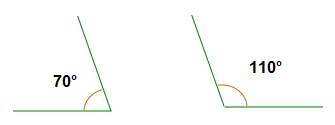
110° and 70° are supplementary. 110 + 70° = 180°
Example
(2.1)
What angle is supplementary with 126°?
Solution
180° − 126° = 54° , 126° + 54° = 180°
The angle 54° is supplementary with the angle 126°.
Vertically Opposite Angles
Vertically opposite angles, which can also referred to as vertical angles.
Are 2 angles of equal size, that are formed between opposite sides of 2 straight lines which intersect each other.
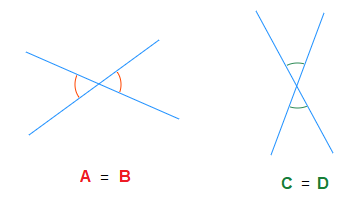
Vertical Angles Theorem
This is a theorem that happens to be a type of proof with regards to the value of angles that are vertically opposite.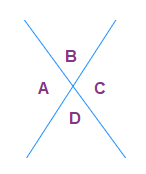
In the image above angles A and B are supplementary with each other, they add up to 180°.
A + B = 180°
Angles B and C are also supplementary angles with each other.
B + C = 180°
A + B = B + C
Now from this stage, using a bit of Algebra.
A = B + C − B => A = C
The same approach can be also followed for the equality of angles B and D.