Transforming Graphs of Functions,
Quadratic Case

Transforming graphs of functions is something that can be done in Math.
Where we already have the graph of a function, but can apply operations to the function in order to change the location or shape of the graph.

This page will show examples of transformations that can be done to a quadratic graph, and the operations that create them.

The quadratic graph we’ll use is a standard curve shown below, but the transformations shown on this page do also apply to other quadratic graphs that can be encountered.

---

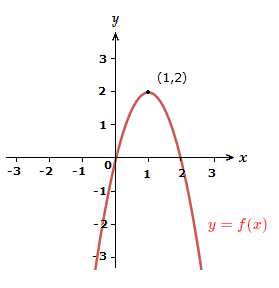
Transforming Graphs of Functions
Examples

1)     $y = \space {\text{-}}f(x)$

Reflect the graph in the  $x$-axis.

2)     $y = \space f({\text{-}}x)$

Reflect the graph in the  $y$-axis.

3)     $y = \space {\text{-}}f({\text{-}}x)$

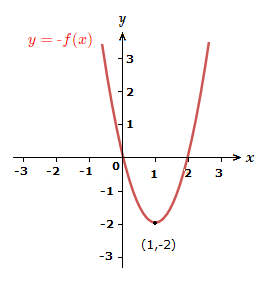
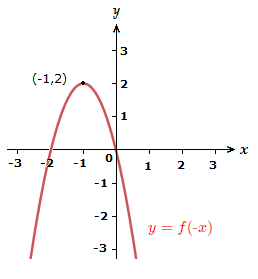
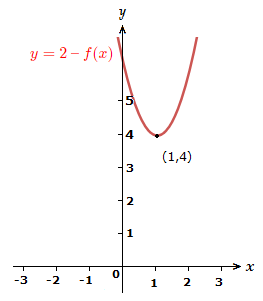
Reflect the graph in the  y-axis, then the  $x$-axis.

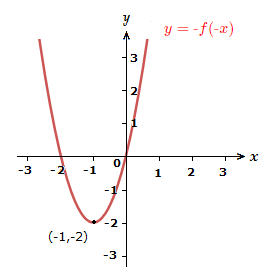
4)     $y = \space f(x) + 1$

Move the graph up the  $x$-axis  1 unit.

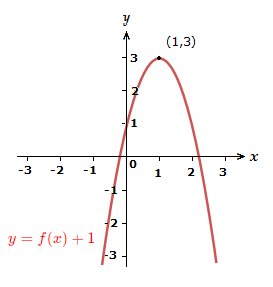
5)     $y = \space f(x + 1)$

Move the graph along the  $y$-axis  1 unit left.

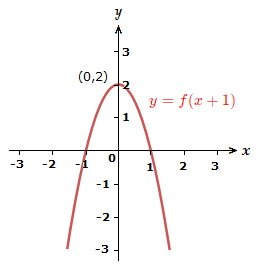
6)     $y = \space f(\frac{x}{2})$

Stretch the graph by 2 in the  $x$  direction.

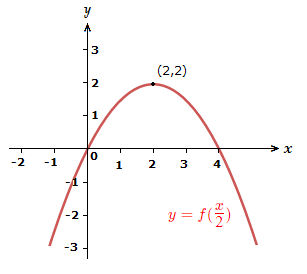
7)     $y = \space 2f(x)$

Stretch the graph by 2 in the  $y$  direction.

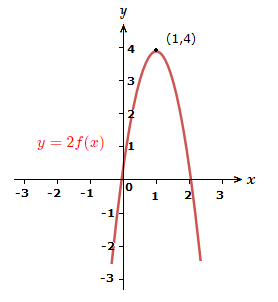
8)     $y = \space 2 \space {\text{--}} \space f(x)$

Reflect the graph in the  $x$-axis,  then move up by 2 units.

1. Home
 ›
2. Algebra 1
› Transforming Graphs of Functions

---

Return to TOP of page