After becoming comfortable with linear equations, learning about quadratic equations and about the roots of a quadratic equation are a good next step in Math.
Quadratic equations have the general form: \color{blue}{ax^{\tt{2}} + bx + c = 0}
The leading term having a variable with an exponent of 2 is what makes the equation a quadratic.
We can’t have a variable with an exponent greater than 2 in a quadratic equation.
So something like x^{\tt{3}} or x^{\tt{5}} cannot be present.
Quadratic Equation Examples:
1) 3x^{\tt{2}} + 2x + 5 = 0
Here, a = 3 , b = 2 , c = 5.
2) x^{\tt{2}} \space {\text{--}} \space 4x + 11 = 0
Here, a = 1, we just don’t write the number beside the variable when it’s only 1.
b = –4 , c = 11.
3) x^{\tt{2}} + 6 = 0
This is still a quadratic equation, it’s just the case that b is zero.
a = 1 , b = 0 , c = 6.
4) {\text{-}}x^{\tt{2}} + 7x = 0
Like example 3), this is a quadratic equation, but now c is zero.
a = –1 , b = 7 , c = 0.
Quadratic Equation Graphs:
The graph of a quadratic is a symmetrical curve called a parabola, this curve can have a maximum or minimum turning point.
We can draw the graph of a quadratic when it is a function in the form of “y =” or “f(x) =“.
1) We can consider the quadratic function, y = x^{\tt{2}} + 2x \space {\text{--}} \space 3.
The graph of this quadratic is shown below.

The curve this quadratic produces has a minimum turning point at (-1,-4).
2) Now we can look a different quadratic function, y = {\text{-}}x^{\tt{2}} + 2x + 3, and observe its graph.
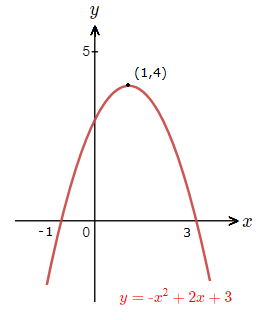
This quadratic produces a curve that has a maximum turning point at (1,4).
It’s actually the general case with quadratic graphs.
If a > 0, then the curve has a minimum turning point.
If a < 0, then the curve has a maximum turning point.
Roots of a Quadratic Equation
The roots of a quadratic equation such as x^{\tt{2}} + 2x \space {\text{--}} \space 3 = 0.
Are the x values/solutions for the equation being equal to zero.
Hence the roots of quadratic equations can also be called ‘zeroes’.
They are the values where the curve of the quadratic touches or crosses the x-axis on a graph.
For a quadratic equation there can be two roots, one root, or sometimes no roots.
More information on solving quadratic equations and finding the roots can be viewed on the solving quadratics by factoring page.