Angles that are coterminal in Math, share the same initial side and the same terminal side between them.
Thus before fully concentrating on coterminal angles examples, it’s helpful to understand about the initial and terminal side of an angle.
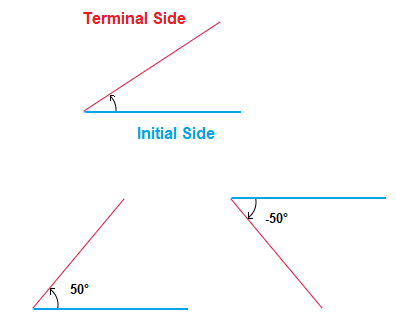
Angles Initial and Terminal Sides
A flat 2D angle created between 2 straight lines, will have one initial side, and one terminal side.
The initial side is the side for beginning the angle measurement,
and the terminal side is the side for ending the angle measurement.
On a standard 2D Cartesian Coordinate axis, the initial side for an angle is often the line in the position of the positive x-axis.
With the end point/vertex of the angle being located at the point (0,0).
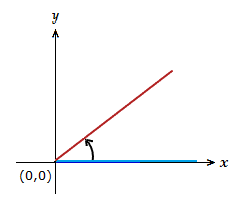
The angle pictured above is measured starting from the blue line on the x-axis that is the initial side, and ends at the red line that is the terminal side.
Coterminal Angles Examples
As referred to at the beginning of the page, angles that are coterminal in Math are angles that share the same initial side and terminal side.
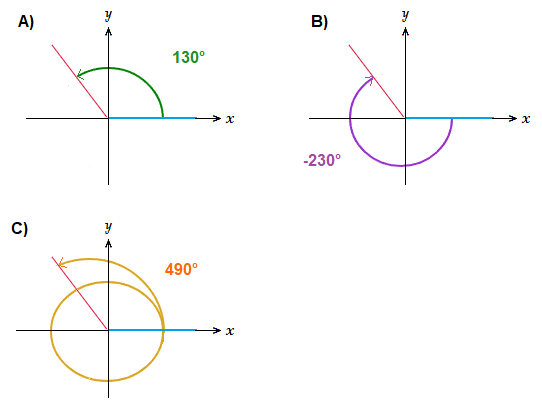
A = 130° , B = –230° , C = 490°
Each angle above, A, B and C above, despite being different sizes,
begin at the same line/side as one another, and also end at the same line/side.
Thus they are angles that are coterminal with each other.
Coterminal Angles Relationships
The relationship between these coterminal angles is that they differ in size by 360°. 130° − 360° = -230° , 130° + 360° = 490°So anytime we wish to obtain an angle that is coterminal to another angle, we just add or subtract 360°.
For example with an angle of size 50°.50° + 360° = 410° , 50° − 360° = –310°
–310° , 50° and 410° are angles that are all coterminal with each other.
In fact an infinite amount of potential coterminal angles that can share the same initial and terminal sides.
As if we wished, we could go around a circle by 360° in either a positive or a negative direction as many times as we like, to find a coterminal angle.
Coterminal Angles and Radians
Coterminal classification with angles also works the same way when dealing with radian measurement of angles as they do with degrees.
Though in this situation, it is 2π that is added or subtracted to an initial angle size, rather than 360°.
Because of the fact that with radian measure, 2π = 360°.
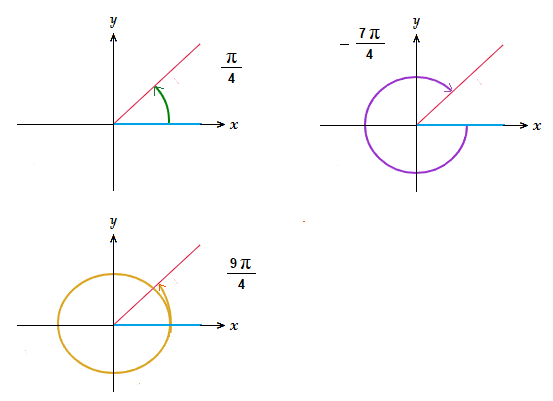
The angles above are coterminal in radian measurement. \boldsymbol{\frac{\pi}{4}} , –\boldsymbol{\frac{7\pi}{4}} , \boldsymbol{\frac{9\pi}{4}}
\boldsymbol{\frac{\pi}{4}} + 2π = \boldsymbol{\frac{9\pi}{4}} , \boldsymbol{\frac{\pi}{4}} − 2π = –\boldsymbol{\frac{7\pi}{4}}
Examples
(1.1)
For an initial angle of θ = 140°.
Find one positive coterminal angle and one negative coterminal angle.
Solution
We just need to do the sums θ + 360° AND θ − 360°.
This will give us one positive and one negative coterminal angle with 140°.
140° + 360° = 500°
140° − 360° = –220°
Angles 500° and –220° are coterminal with 140°.
(1.2)
With an initial angle of θ = \boldsymbol{\frac{4\pi}{5}},
find one positive coterminal angle and one negative coterminal angle.
Solution
As θ here is given in radian measure.
We look to perform θ + 2π AND θ − 2π
That will result in a positive and a negative coterminal angle with \boldsymbol{\frac{4\pi}{5}}.
\boldsymbol{\frac{4\pi}{5}} + 2π = \boldsymbol{\frac{4\pi}{5}} + \bf{\frac{10\pi}{5}} = \boldsymbol{\frac{14\pi}{5}}
\boldsymbol{\frac{4\pi}{5}} − 2π = \boldsymbol{\frac{4\pi}{5}} − \bf{\frac{10\pi}{5}} = –\boldsymbol{\frac{6\pi}{5}}
Angles \boldsymbol{\frac{14\pi}{5}} and –\boldsymbol{\frac{6\pi}{5}} are coterminal with \boldsymbol{\frac{2\pi}{5}}.
(1.3)
Starting with an initial angle of θ = 60°.
Find two negative angles that are coterminal.
Solution
To obtain two negative angles that are coterminal, we will subtract 360° twice from 60°.
A subtracting 360° twice will result in two negative angles that are coterminal.
60° − 360° = –300°
60° − 360° − 360° = –660°
–300° and –660° are coterminal with 60°